
Here’s an amortization factor rate calculator to help you compute for monthly payments quickly. Want to find the amortization factor for your home loan? I tabulated them all for you here. Check them out below.
Amortization Factor Rate Calculator
What is factor rate?
The amortization factor rate is used to easily compute for monthly amortization payments. We already tabulated amortization factors for mortgage/home loan interest rates ranging from 1% to 20% per year, with payment terms ranging from 1 to 30 years to pay.
How to use amortization factor rates
To calculate the monthly amortization, just multiply the loan amount with the amortization factor rate for the corresponding interest rate and term (in years) in the applicable table below. The resulting monthly amortization is a combination of principal and interest.
Terms of use: A friendly reminder for copycats…
We have noticed a lot of copycat sites out there (yes, we know who you are!) who plagiarize our content just like the amortization factor rate tables below. Because of this, we have added data within the tables which we use to track copied content. This is visible even if the tables are converted into images.
If you want to use our content, you may do so with proper attribution (with a link that leads back to this post), otherwise stop plagiarizing our content… copyright violators shall be dealt with accordingly (Hint: Google also knows who are the copy cats).
How to compute for Amortization Factor Rate
To compute for amortization factor rate, all you need is the annual interest rate and loan term, which you can enter into the amortization factor rate calculator I created above. Just enter the loan term (in years) and the interest rate (annual), and it will auto-compute for the amortization factor.
Use this calculator if your loan term is not typical, and is not found in the tables below.
The formula I used for my calculator can be seen below. For a detailed explanation and examples, you may refer to my article: How To Compute For Amortization Factor.
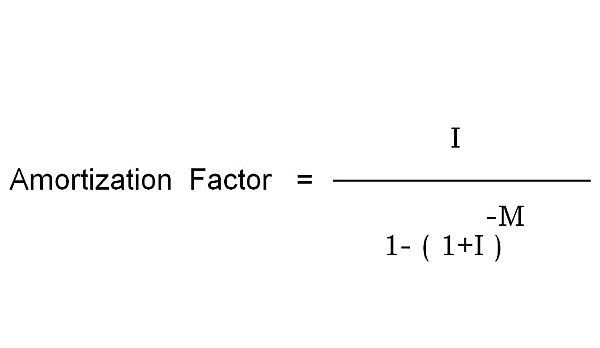
Where:
I = the monthly interest rate. You can easily get this by dividing the annual interest rate by 12
M = the loan payment term in months. Just multiply the loan term by 12.
The basis for this formula, which includes the derivation can be found in this wikipedia article on amortization.
Quicklinks to typical amortization factor rates
- 1% to 2.75%
- 3% to 4.75%
- 5% to 6.75%
- 7% to 8.75%
- 9% to 10.75%
- 11% to 12.75%
- 13% to 14.75%
- 15% to 16.75%
- 17% to 18.75%
- 19% to 20.75%
- Sample Computation
Amortization factor rate tables
(You can scroll/swipe left or right when viewing from a small screen)
1.00% to 2.75%
| Term (years) | 1.00% | 1.25% | 1.50% | 1.75% | 2.00% | 2.25% | 2.50% | 2.75% |
|---|---|---|---|---|---|---|---|---|
| 1 | 0.0837854116 | 0.0838986464 | 0.0840119673 | 0.0841253743 | 0.0842388673 | 0.0843524462 | 0.0844661112 | 0.0845798620 |
| 2 | 0.0421020803 | 0.0422113666 | 0.0423208259 | 0.0424304582 | 0.0425402634 | 0.0426502414 | 0.0427603922 | 0.0428707158 |
| 3 | 0.0282080993 | 0.0283163296 | 0.0284248197 | 0.0285335694 | 0.0286425787 | 0.0287518475 | 0.0288613757 | 0.0289711631 |
| 4 | 0.0212614559 | 0.0213693533 | 0.0214775972 | 0.0215861873 | 0.0216951236 | 0.0218044059 | 0.0219140340 | 0.0220240078 |
| 5 | 0.0170937474 | 0.0172016012 | 0.0173098881 | 0.0174186077 | 0.0175277601 | 0.0176373448 | 0.0177473616 | 0.0178578103 |
| 6 | 0.0143155065 | 0.0144234612 | 0.0145319355 | 0.0146409292 | 0.0147504419 | 0.0148604733 | 0.0149710230 | 0.0150820906 |
| 7 | 0.0123312469 | 0.0124393851 | 0.0125481295 | 0.0126574796 | 0.0127674350 | 0.0128779953 | 0.0129891600 | 0.0131009284 |
| 8 | 0.0108432257 | 0.0109515990 | 0.0110606649 | 0.0111704229 | 0.0112808724 | 0.0113920128 | 0.0115038433 | 0.0116163633 |
| 9 | 0.0096860300 | 0.0097946728 | 0.0099040945 | 0.0100142946 | 0.0101252722 | 0.0102370266 | 0.0103495567 | 0.0104628616 |
| 10 | 0.0087604121 | 0.0088693484 | 0.0089791500 | 0.0090898160 | 0.0092013454 | 0.0093137372 | 0.0094269902 | 0.0095411031 |
| 11 | 0.0080032145 | 0.0081124617 | 0.0082226604 | 0.0083338095 | 0.0084459079 | 0.0085589540 | 0.0086729464 | 0.0087878835 |
| 12 | 0.0073723321 | 0.0074819032 | 0.0075925120 | 0.0077041571 | 0.0078168369 | 0.0079305498 | 0.0080452938 | 0.0081610670 |
| 13 | 0.0068386151 | 0.0069485202 | 0.0070595490 | 0.0071716997 | 0.0072849707 | 0.0073993596 | 0.0075148643 | 0.0076314820 |
| 14 | 0.0063812424 | 0.0064914893 | 0.0066029457 | 0.0067156098 | 0.0068294792 | 0.0069445513 | 0.0070608232 | 0.0071782919 |
| 15 | 0.0059849451 | 0.0060955400 | 0.0062074302 | 0.0063206135 | 0.0064350870 | 0.0065508477 | 0.0066678921 | 0.0067862164 |
| 16 | 0.0056382716 | 0.0057492194 | 0.0058615483 | 0.0059752555 | 0.0060903377 | 0.0062067912 | 0.0063246118 | 0.0064437950 |
| 17 | 0.0053324646 | 0.0054437695 | 0.0055565411 | 0.0056707760 | 0.0057864704 | 0.0059036198 | 0.0060222194 | 0.0061422638 |
| 18 | 0.0050607131 | 0.0051723785 | 0.0052855959 | 0.0054003616 | 0.0055166709 | 0.0056345187 | 0.0057538993 | 0.0058748063 |
| 19 | 0.0048176398 | 0.0049296685 | 0.0050433345 | 0.0051586334 | 0.0052755598 | 0.0053941078 | 0.0055142707 | 0.0056360412 |
| 20 | 0.0045989431 | 0.0047113373 | 0.0048254541 | 0.0049412881 | 0.0050588334 | 0.0051780829 | 0.0052990289 | 0.0054216631 |
| 21 | 0.0044011404 | 0.0045139023 | 0.0046284716 | 0.0047448424 | 0.0048630077 | 0.0049829595 | 0.0051046891 | 0.0052281867 |
| 22 | 0.0042213826 | 0.0043345137 | 0.0044495371 | 0.0045664460 | 0.0046852323 | 0.0048058870 | 0.0049284001 | 0.0050527604 |
| 23 | 0.0040573160 | 0.0041708178 | 0.0042862965 | 0.0044037444 | 0.0045231523 | 0.0046445101 | 0.0047678064 | 0.0048930284 |
| 24 | 0.0039069792 | 0.0040208529 | 0.0041367879 | 0.0042547755 | 0.0043748055 | 0.0044968663 | 0.0046209451 | 0.0047470275 |
| 25 | 0.0037687245 | 0.0038829711 | 0.0039993633 | 0.0041178912 | 0.0042385434 | 0.0043613070 | 0.0044861673 | 0.0046131086 |
| 26 | 0.0036411579 | 0.0037557782 | 0.0038726282 | 0.0039916968 | 0.0041129712 | 0.0042364370 | 0.0043620779 | 0.0044898761 |
| 27 | 0.0035230917 | 0.0036380866 | 0.0037553949 | 0.0038750044 | 0.0039969009 | 0.0041210682 | 0.0042474883 | 0.0043761413 |
| 28 | 0.0034135081 | 0.0035288780 | 0.0036466450 | 0.0037667956 | 0.0038893138 | 0.0040141818 | 0.0041413796 | 0.0042708851 |
| 29 | 0.0033115294 | 0.0034272750 | 0.0035455010 | 0.0036661925 | 0.0037893321 | 0.0039148998 | 0.0040428736 | 0.0041732291 |
| 30 | 0.0032163952 | 0.0033325168 | 0.0034512021 | 0.0035724345 | 0.0036961947 | 0.0038224610 | 0.0039512090 | 0.0040824118 |
3.00% to 4.75%
| Term (years) | 3.00% | 3.25% | 3.50% | 3.75% | 4.00% | 4.25% | 4.50% | 4.75% |
|---|---|---|---|---|---|---|---|---|
| 1 | 0.0846936988 | 0.0848076214 | 0.0849216298 | 0.0850357241 | 0.0851499042 | 0.0852641700 | 0.0853785216 | 0.0854929589 |
| 2 | 0.0429812120 | 0.0430918808 | 0.0432027221 | 0.0433137360 | 0.0434249222 | 0.0435362807 | 0.0436478116 | 0.0437595146 |
| 3 | 0.0290812096 | 0.0291915152 | 0.0293020797 | 0.0294129030 | 0.0295239850 | 0.0296353255 | 0.0297469245 | 0.0298587817 |
| 4 | 0.0221343270 | 0.0222449915 | 0.0223560011 | 0.0224673555 | 0.0225790546 | 0.0226910982 | 0.0228034861 | 0.0229162179 |
| 5 | 0.0179686907 | 0.0180800023 | 0.0181917450 | 0.0183039183 | 0.0184165221 | 0.0185295558 | 0.0186430192 | 0.0187569120 |
| 6 | 0.0151936758 | 0.0153057781 | 0.0154183971 | 0.0155315322 | 0.0156451831 | 0.0157593491 | 0.0158740297 | 0.0159892245 |
| 7 | 0.0132133001 | 0.0133262744 | 0.0134398506 | 0.0135540282 | 0.0136688063 | 0.0137841843 | 0.0139001613 | 0.0140167365 |
| 8 | 0.0117295719 | 0.0118434684 | 0.0119580517 | 0.0120733210 | 0.0121892753 | 0.0123059135 | 0.0124232344 | 0.0125412370 |
| 9 | 0.0105769404 | 0.0106917917 | 0.0108074144 | 0.0109238073 | 0.0110409689 | 0.0111588978 | 0.0112775926 | 0.0113970516 |
| 10 | 0.0096560745 | 0.0097719029 | 0.0098885867 | 0.0100061243 | 0.0101245138 | 0.0102437533 | 0.0103638409 | 0.0104847743 |
| 11 | 0.0089037635 | 0.0090205845 | 0.0091383444 | 0.0092570409 | 0.0093766719 | 0.0094972347 | 0.0096187269 | 0.0097411457 |
| 12 | 0.0082778669 | 0.0083956912 | 0.0085145374 | 0.0086344025 | 0.0087552837 | 0.0088771778 | 0.0090000816 | 0.0091239916 |
| 13 | 0.0077492100 | 0.0078680454 | 0.0079879847 | 0.0081090247 | 0.0082311616 | 0.0083543914 | 0.0084787102 | 0.0086041136 |
| 14 | 0.0072969539 | 0.0074168055 | 0.0075378427 | 0.0076600612 | 0.0077834566 | 0.0079080241 | 0.0080337585 | 0.0081606547 |
| 15 | 0.0069058164 | 0.0070266877 | 0.0071488254 | 0.0072722244 | 0.0073968793 | 0.0075227841 | 0.0076499329 | 0.0077783192 |
| 16 | 0.0065643358 | 0.0066862288 | 0.0068094684 | 0.0069340483 | 0.0070599619 | 0.0071872024 | 0.0073157624 | 0.0074456343 |
| 17 | 0.0062637470 | 0.0063866629 | 0.0065110044 | 0.0066367643 | 0.0067639349 | 0.0068925079 | 0.0070224746 | 0.0071538261 |
| 18 | 0.0059972329 | 0.0061211716 | 0.0062466143 | 0.0063735524 | 0.0065019770 | 0.0066318782 | 0.0067632461 | 0.0068960699 |
| 19 | 0.0057594112 | 0.0058843720 | 0.0060109142 | 0.0061390280 | 0.0062687027 | 0.0063999270 | 0.0065326893 | 0.0066669771 |
| 20 | 0.0055459760 | 0.0056719576 | 0.0057995972 | 0.0059288831 | 0.0060598033 | 0.0061923447 | 0.0063264938 | 0.0064622363 |
| 21 | 0.0053534415 | 0.0054804421 | 0.0056091761 | 0.0057396303 | 0.0058717906 | 0.0060056423 | 0.0061411698 | 0.0062783569 |
| 22 | 0.0051789557 | 0.0053069730 | 0.0054367980 | 0.0055684158 | 0.0057018104 | 0.0058369649 | 0.0059738617 | 0.0061124823 |
| 23 | 0.0050201624 | 0.0051491936 | 0.0052801058 | 0.0054128821 | 0.0055475044 | 0.0056839537 | 0.0058222101 | 0.0059622527 |
| 24 | 0.0048750980 | 0.0050051399 | 0.0051371351 | 0.0052710644 | 0.0054069075 | 0.0055446430 | 0.0056842485 | 0.0058257007 |
| 25 | 0.0047421131 | 0.0048731623 | 0.0050062357 | 0.0051413120 | 0.0052783684 | 0.0054173810 | 0.0055583248 | 0.0057011736 |
| 26 | 0.0046198119 | 0.0047518645 | 0.0048860112 | 0.0050222282 | 0.0051604900 | 0.0053007701 | 0.0054430407 | 0.0055872727 |
| 27 | 0.0045070054 | 0.0046400573 | 0.0047752720 | 0.0049126229 | 0.0050520818 | 0.0051936194 | 0.0053372049 | 0.0054828061 |
| 28 | 0.0044026742 | 0.0045367212 | 0.0046729982 | 0.0048114759 | 0.0049521234 | 0.0050949080 | 0.0052397960 | 0.0053867519 |
| 29 | 0.0043059398 | 0.0044409771 | 0.0045783105 | 0.0047179077 | 0.0048597347 | 0.0050037555 | 0.0051499332 | 0.0052982290 |
| 30 | 0.0042160403 | 0.0043520632 | 0.0044904469 | 0.0046311559 | 0.0047741530 | 0.0049193989 | 0.0050668531 | 0.0052164734 |
5.00% to 6.75%
| Term (years) | 5.00% | 5.25% | 5.50% | 5.75% | 6.00% | 6.25% | 6.50% | 6.75% |
|---|---|---|---|---|---|---|---|---|
| 1 | 0.0856074818 | 0.0857220904 | 0.0858367846 | 0.0859515644 | 0.0860664297 | 0.0861813806 | 0.0862964170 | 0.0864115388 |
| 2 | 0.0438713897 | 0.0439834370 | 0.0440956562 | 0.0442080473 | 0.0443206103 | 0.0444333450 | 0.0445462514 | 0.0446593295 |
| 3 | 0.0299708971 | 0.0300832705 | 0.0301959018 | 0.0303087908 | 0.0304219375 | 0.0305353415 | 0.0306490029 | 0.0307629214 |
| 4 | 0.0230292936 | 0.0231427128 | 0.0232564752 | 0.0233705807 | 0.0234850290 | 0.0235998199 | 0.0237149529 | 0.0238304280 |
| 5 | 0.0188712336 | 0.0189859838 | 0.0191011622 | 0.0192167682 | 0.0193328015 | 0.0194492617 | 0.0195661482 | 0.0196834607 |
| 6 | 0.0161049327 | 0.0162211537 | 0.0163378871 | 0.0164551320 | 0.0165728879 | 0.0166911540 | 0.0168099296 | 0.0169292140 |
| 7 | 0.0141339091 | 0.0142516781 | 0.0143700427 | 0.0144890018 | 0.0146085545 | 0.0147286997 | 0.0148494365 | 0.0149707636 |
| 8 | 0.0126599200 | 0.0127792822 | 0.0128993222 | 0.0130200387 | 0.0131414302 | 0.0132634954 | 0.0133862326 | 0.0135096404 |
| 9 | 0.0115172732 | 0.0116382556 | 0.0117599971 | 0.0118824957 | 0.0120057496 | 0.0121297568 | 0.0122545151 | 0.0123800224 |
| 10 | 0.0106065515 | 0.0107291701 | 0.0108526278 | 0.0109769220 | 0.0111020502 | 0.0112280097 | 0.0113547977 | 0.0114824114 |
| 11 | 0.0098644882 | 0.0099887516 | 0.0101139326 | 0.0102400281 | 0.0103670346 | 0.0104949488 | 0.0106237671 | 0.0107534858 |
| 12 | 0.0092489041 | 0.0093748155 | 0.0095017217 | 0.0096296187 | 0.0097585021 | 0.0098883677 | 0.0100192109 | 0.0101510269 |
| 13 | 0.0087305970 | 0.0088581558 | 0.0089867851 | 0.0091164798 | 0.0092472344 | 0.0093790437 | 0.0095119019 | 0.0096458031 |
| 14 | 0.0082887071 | 0.0084179099 | 0.0085482571 | 0.0086797423 | 0.0088123592 | 0.0089461010 | 0.0090809608 | 0.0092169314 |
| 15 | 0.0079079363 | 0.0080387772 | 0.0081708345 | 0.0083041009 | 0.0084385683 | 0.0085742287 | 0.0087110737 | 0.0088490946 |
| 16 | 0.0075768100 | 0.0077092811 | 0.0078430390 | 0.0079780747 | 0.0081143786 | 0.0082519413 | 0.0083907527 | 0.0085308025 |
| 17 | 0.0072865528 | 0.0074206447 | 0.0075560917 | 0.0076928831 | 0.0078310077 | 0.0079704543 | 0.0081112110 | 0.0082532659 |
| 18 | 0.0070303385 | 0.0071660404 | 0.0073031636 | 0.0074416954 | 0.0075816232 | 0.0077229336 | 0.0078656129 | 0.0080096470 |
| 19 | 0.0068027775 | 0.0069400772 | 0.0070788622 | 0.0072191181 | 0.0073608299 | 0.0075039824 | 0.0076485597 | 0.0077945457 |
| 20 | 0.0065995574 | 0.0067384417 | 0.0068788731 | 0.0070208351 | 0.0071643106 | 0.0073092820 | 0.0074557314 | 0.0076036401 |
| 21 | 0.0064171865 | 0.0065576411 | 0.0066997024 | 0.0068433516 | 0.0069885692 | 0.0071353352 | 0.0072836293 | 0.0074334304 |
| 22 | 0.0062528075 | 0.0063948174 | 0.0065384911 | 0.0066838075 | 0.0068307445 | 0.0069792797 | 0.0071293899 | 0.0072810517 |
| 23 | 0.0061040597 | 0.0062476088 | 0.0063928766 | 0.0065398392 | 0.0066884720 | 0.0068387498 | 0.0069906467 | 0.0071441364 |
| 24 | 0.0059689751 | 0.0061140467 | 0.0062608895 | 0.0064094766 | 0.0065597807 | 0.0067117736 | 0.0068654266 | 0.0070207106 |
| 25 | 0.0058459004 | 0.0059924772 | 0.0061408749 | 0.0062910640 | 0.0064430140 | 0.0065966938 | 0.0067520716 | 0.0069091153 |
| 26 | 0.0057334362 | 0.0058815000 | 0.0060314323 | 0.0061832001 | 0.0063367699 | 0.0064921076 | 0.0066491781 | 0.0068079460 |
| 27 | 0.0056303900 | 0.0057799223 | 0.0059313678 | 0.0060846905 | 0.0062398535 | 0.0063968193 | 0.0065555497 | 0.0067160061 |
| 28 | 0.0055357395 | 0.0056867211 | 0.0058396582 | 0.0059945113 | 0.0061512402 | 0.0063098038 | 0.0064701608 | 0.0066322691 |
| 29 | 0.0054486030 | 0.0056010143 | 0.0057554207 | 0.0059117792 | 0.0060700461 | 0.0062301769 | 0.0063921267 | 0.0065558498 |
| 30 | 0.0053682162 | 0.0055220370 | 0.0056778900 | 0.0058357286 | 0.0059955053 | 0.0061571720 | 0.0063206802 | 0.0064859810 |
7.00% to 8.75%
| Term (years) | 7.00% | 7.25% | 7.50% | 7.75% | 8.00% | 8.25% | 8.50% | 8.75% |
|---|---|---|---|---|---|---|---|---|
| 1 | 0.0865267461 | 0.0866420388 | 0.0867574169 | 0.0868728803 | 0.0869884291 | 0.0871040631 | 0.0872197825 | 0.0873355870 |
| 2 | 0.0447725791 | 0.0448860002 | 0.0449995927 | 0.0451133564 | 0.0452272915 | 0.0453413976 | 0.0454556749 | 0.0455701231 |
| 3 | 0.0308770969 | 0.0309915292 | 0.0311062182 | 0.0312211636 | 0.0313363655 | 0.0314518234 | 0.0315675374 | 0.0316835072 |
| 4 | 0.0239462447 | 0.0240624028 | 0.0241789019 | 0.0242957419 | 0.0244129223 | 0.0245304429 | 0.0246483034 | 0.0247665033 |
| 5 | 0.0198011985 | 0.0199193614 | 0.0200379486 | 0.0201569598 | 0.0202763943 | 0.0203962517 | 0.0205165313 | 0.0206372327 |
| 6 | 0.0170490065 | 0.0171693062 | 0.0172901123 | 0.0174114241 | 0.0175332406 | 0.0176555611 | 0.0177783846 | 0.0179017103 |
| 7 | 0.0150926800 | 0.0152151845 | 0.0153382759 | 0.0154619529 | 0.0155862144 | 0.0157110590 | 0.0158364854 | 0.0159624923 |
| 8 | 0.0136337171 | 0.0137584610 | 0.0138838706 | 0.0140099439 | 0.0141366793 | 0.0142640748 | 0.0143921286 | 0.0145208387 |
| 9 | 0.0125062766 | 0.0126332753 | 0.0127610162 | 0.0128894969 | 0.0130187149 | 0.0131486677 | 0.0132793527 | 0.0134107673 |
| 10 | 0.0116108479 | 0.0117401041 | 0.0118701769 | 0.0120010631 | 0.0121327594 | 0.0122652625 | 0.0123985689 | 0.0125326750 |
| 11 | 0.0108841009 | 0.0110156087 | 0.0111480051 | 0.0112812859 | 0.0114154469 | 0.0115504837 | 0.0116863919 | 0.0118231668 |
| 12 | 0.0102838110 | 0.0104175581 | 0.0105522631 | 0.0106879208 | 0.0108245258 | 0.0109620727 | 0.0111005556 | 0.0112399690 |
| 13 | 0.0097807414 | 0.0099167106 | 0.0100537042 | 0.0101917159 | 0.0103307388 | 0.0104707663 | 0.0106117912 | 0.0107538066 |
| 14 | 0.0093540054 | 0.0094921753 | 0.0096314334 | 0.0097717717 | 0.0099131820 | 0.0100556560 | 0.0101991854 | 0.0103437613 |
| 15 | 0.0089882827 | 0.0091286288 | 0.0092701236 | 0.0094127575 | 0.0095565208 | 0.0097014036 | 0.0098473956 | 0.0099944865 |
| 16 | 0.0086720802 | 0.0088145750 | 0.0089582759 | 0.0091031715 | 0.0092492503 | 0.0093965004 | 0.0095449100 | 0.0096944668 |
| 17 | 0.0083966065 | 0.0085412202 | 0.0086870939 | 0.0088342145 | 0.0089825684 | 0.0091321419 | 0.0092829210 | 0.0094348915 |
| 18 | 0.0081550217 | 0.0083017221 | 0.0084497333 | 0.0085990399 | 0.0087496262 | 0.0089014765 | 0.0090545745 | 0.0092089040 |
| 19 | 0.0079419238 | 0.0080906770 | 0.0082407882 | 0.0083922398 | 0.0085450138 | 0.0086990921 | 0.0088544563 | 0.0090110878 |
| 20 | 0.0077529894 | 0.0079037598 | 0.0080559319 | 0.0082094856 | 0.0083644007 | 0.0085206565 | 0.0086782323 | 0.0088371071 |
| 21 | 0.0075847171 | 0.0077374678 | 0.0078916601 | 0.0080472716 | 0.0082042796 | 0.0083626608 | 0.0085223921 | 0.0086834497 |
| 22 | 0.0074342410 | 0.0075889334 | 0.0077451040 | 0.0079027277 | 0.0080617791 | 0.0082222323 | 0.0083840616 | 0.0085472406 |
| 23 | 0.0072991922 | 0.0074557868 | 0.0076138926 | 0.0077734817 | 0.0079345259 | 0.0080969967 | 0.0082608654 | 0.0084261033 |
| 24 | 0.0071775958 | 0.0073360521 | 0.0074960490 | 0.0076575557 | 0.0078205412 | 0.0079849742 | 0.0081508232 | 0.0083180568 |
| 25 | 0.0070677920 | 0.0072280686 | 0.0073899118 | 0.0075532876 | 0.0077181622 | 0.0078845013 | 0.0080522708 | 0.0082214364 |
| 26 | 0.0069683756 | 0.0071304306 | 0.0072940744 | 0.0074592703 | 0.0076259811 | 0.0077941700 | 0.0079637998 | 0.0081348334 |
| 27 | 0.0068781493 | 0.0070419400 | 0.0072073384 | 0.0073743046 | 0.0075427986 | 0.0077127805 | 0.0078842103 | 0.0080570480 |
| 28 | 0.0067960861 | 0.0069615694 | 0.0071286759 | 0.0072973626 | 0.0074675864 | 0.0076393045 | 0.0078124739 | 0.0079870520 |
| 29 | 0.0067213006 | 0.0068884330 | 0.0070572008 | 0.0072275579 | 0.0073994581 | 0.0075728556 | 0.0077477046 | 0.0079239597 |
| 30 | 0.0066530250 | 0.0068217628 | 0.0069921451 | 0.0071641225 | 0.0073376457 | 0.0075126660 | 0.0076891348 | 0.0078670041 |
9.00% to 10.75%
| Term (years) | 9.00% | 9.25% | 9.50% | 9.75% | 10.00% | 10.25% | 10.50% | 10.75% |
|---|---|---|---|---|---|---|---|---|
| 1 | 0.0874514768 | 0.0875674517 | 0.0876835118 | 0.0877996570 | 0.0879158872 | 0.0880322026 | 0.0881486029 | 0.0882650882 |
| 2 | 0.0456847423 | 0.0457995323 | 0.0459144930 | 0.0460296244 | 0.0461449263 | 0.0462603988 | 0.0463760416 | 0.0464918548 |
| 3 | 0.0317997327 | 0.0319162136 | 0.0320329497 | 0.0321499410 | 0.0322671872 | 0.0323846881 | 0.0325024435 | 0.0326204532 |
| 4 | 0.0248850424 | 0.0250039203 | 0.0251231367 | 0.0252426912 | 0.0253625834 | 0.0254828131 | 0.0256033798 | 0.0257242831 |
| 5 | 0.0207583552 | 0.0208798983 | 0.0210018613 | 0.0211242437 | 0.0212470447 | 0.0213702638 | 0.0214939004 | 0.0216179537 |
| 6 | 0.0180255372 | 0.0181498643 | 0.0182746908 | 0.0184000156 | 0.0185258378 | 0.0186521562 | 0.0187789700 | 0.0189062779 |
| 7 | 0.0160890783 | 0.0162162419 | 0.0163439817 | 0.0164722962 | 0.0166011840 | 0.0167306435 | 0.0168606731 | 0.0169912713 |
| 8 | 0.0146502033 | 0.0147802202 | 0.0149108873 | 0.0150422027 | 0.0151741641 | 0.0153067693 | 0.0154400162 | 0.0155739024 |
| 9 | 0.0135429087 | 0.0136757741 | 0.0138093608 | 0.0139436658 | 0.0140786862 | 0.0142144190 | 0.0143508612 | 0.0144880096 |
| 10 | 0.0126675774 | 0.0128032722 | 0.0129397558 | 0.0130770242 | 0.0132150737 | 0.0133539002 | 0.0134934997 | 0.0136338680 |
| 11 | 0.0119608039 | 0.0120992985 | 0.0122386455 | 0.0123788402 | 0.0125198775 | 0.0126617522 | 0.0128044593 | 0.0129479933 |
| 12 | 0.0113803070 | 0.0115215634 | 0.0116637324 | 0.0118068075 | 0.0119507826 | 0.0120956512 | 0.0122414068 | 0.0123880428 |
| 13 | 0.0108968051 | 0.0110407794 | 0.0111857219 | 0.0113316250 | 0.0114784809 | 0.0116262818 | 0.0117750196 | 0.0119246863 |
| 14 | 0.0104893750 | 0.0106360177 | 0.0107836800 | 0.0109323529 | 0.0110820269 | 0.0112326925 | 0.0113843401 | 0.0115369600 |
| 15 | 0.0101426658 | 0.0102919229 | 0.0104422468 | 0.0105936266 | 0.0107460512 | 0.0108995092 | 0.0110539892 | 0.0112094798 |
| 16 | 0.0098451584 | 0.0099969723 | 0.0101498957 | 0.0103039157 | 0.0104590193 | 0.0106151933 | 0.0107724244 | 0.0109306991 |
| 17 | 0.0095880390 | 0.0097423489 | 0.0098978065 | 0.0100543967 | 0.0102121046 | 0.0103709149 | 0.0105308122 | 0.0106917813 |
| 18 | 0.0093644484 | 0.0095211908 | 0.0096791145 | 0.0098382023 | 0.0099984370 | 0.0101598012 | 0.0103222776 | 0.0104858485 |
| 19 | 0.0091689678 | 0.0093280771 | 0.0094883967 | 0.0096499072 | 0.0098125891 | 0.0099764229 | 0.0101413890 | 0.0103074675 |
| 20 | 0.0089972596 | 0.0091586683 | 0.0093213119 | 0.0094851685 | 0.0096502165 | 0.0098164339 | 0.0099837989 | 0.0101522895 |
| 21 | 0.0088458102 | 0.0090094494 | 0.0091743436 | 0.0093404685 | 0.0095078001 | 0.0096763141 | 0.0098459865 | 0.0100167930 |
| 22 | 0.0087117432 | 0.0088775429 | 0.0090446133 | 0.0092129279 | 0.0093824600 | 0.0095531831 | 0.0097250708 | 0.0098980967 |
| 23 | 0.0085926815 | 0.0087605709 | 0.0089297426 | 0.0091001675 | 0.0092718166 | 0.0094446611 | 0.0096186723 | 0.0097938214 |
| 24 | 0.0084866433 | 0.0086565511 | 0.0088277487 | 0.0090002046 | 0.0091738873 | 0.0093487657 | 0.0095248088 | 0.0097019856 |
| 25 | 0.0083919636 | 0.0085638184 | 0.0087369666 | 0.0089113742 | 0.0090870075 | 0.0092638328 | 0.0094418171 | 0.0096209272 |
| 26 | 0.0083072338 | 0.0084809641 | 0.0086559878 | 0.0088322683 | 0.0090097696 | 0.0091884559 | 0.0093682918 | 0.0095492424 |
| 27 | 0.0082312540 | 0.0084067888 | 0.0085836133 | 0.0087616887 | 0.0089409766 | 0.0091214390 | 0.0093030385 | 0.0094857381 |
| 28 | 0.0081629964 | 0.0083402652 | 0.0085188167 | 0.0086986098 | 0.0088796040 | 0.0090617591 | 0.0092450357 | 0.0094293953 |
| 29 | 0.0081015759 | 0.0082805088 | 0.0084607143 | 0.0086421492 | 0.0088247707 | 0.0090085368 | 0.0091934063 | 0.0093793387 |
| 30 | 0.0080462262 | 0.0082267543 | 0.0084085421 | 0.0085915441 | 0.0087757157 | 0.0089610130 | 0.0091473929 | 0.0093348136 |
11.00% to 12.75%
| Term (years) | 11.00% | 11.25% | 11.50% | 11.75% | 12.00% | 12.25% | 12.50% | 12.75% |
|---|---|---|---|---|---|---|---|---|
| 1 | 0.0883816585 | 0.0884983137 | 0.0886150539 | 0.0887318789 | 0.0888487887 | 0.0889657833 | 0.0890828627 | 0.0892000269 |
| 2 | 0.0466078382 | 0.0467239917 | 0.0468403153 | 0.0469568088 | 0.0470734722 | 0.0471903054 | 0.0473073082 | 0.0474244807 |
| 3 | 0.0327387171 | 0.0328572349 | 0.0329760064 | 0.0330950315 | 0.0332143098 | 0.0333338413 | 0.0334536256 | 0.0335736626 |
| 4 | 0.0258455226 | 0.0259670980 | 0.0260890089 | 0.0262112548 | 0.0263338354 | 0.0264567503 | 0.0265799989 | 0.0267035809 |
| 5 | 0.0217424231 | 0.0218673079 | 0.0219926074 | 0.0221183209 | 0.0222444477 | 0.0223709870 | 0.0224979382 | 0.0226253005 |
| 6 | 0.0190340790 | 0.0191623721 | 0.0192911562 | 0.0194204300 | 0.0195501925 | 0.0196804425 | 0.0198111787 | 0.0199424000 |
| 7 | 0.0171224364 | 0.0172541668 | 0.0173864608 | 0.0175193167 | 0.0176527328 | 0.0177867073 | 0.0179212384 | 0.0180563244 |
| 8 | 0.0157084257 | 0.0158435836 | 0.0159793738 | 0.0161157939 | 0.0162528414 | 0.0163905138 | 0.0165288086 | 0.0166677233 |
| 9 | 0.0146258610 | 0.0147644123 | 0.0149036603 | 0.0150436015 | 0.0151842326 | 0.0153255503 | 0.0154675510 | 0.0156102313 |
| 10 | 0.0137750011 | 0.0139168947 | 0.0140595444 | 0.0142029459 | 0.0143470948 | 0.0144919867 | 0.0146376169 | 0.0147839809 |
| 11 | 0.0130923490 | 0.0132375210 | 0.0133835037 | 0.0135302915 | 0.0136778788 | 0.0138262599 | 0.0139754290 | 0.0141253803 |
| 12 | 0.0125355526 | 0.0126839292 | 0.0128331658 | 0.0129832556 | 0.0131341914 | 0.0132859662 | 0.0134385728 | 0.0135920040 |
| 13 | 0.0120752736 | 0.0122267733 | 0.0123791769 | 0.0125324761 | 0.0126866622 | 0.0128417267 | 0.0129976607 | 0.0131544557 |
| 14 | 0.0116905423 | 0.0118450770 | 0.0120005542 | 0.0121569637 | 0.0123142953 | 0.0124725387 | 0.0126316835 | 0.0127917194 |
| 15 | 0.0113659693 | 0.0115234460 | 0.0116818981 | 0.0118413136 | 0.0120016806 | 0.0121629871 | 0.0123252208 | 0.0124883698 |
| 16 | 0.0110900040 | 0.0112503254 | 0.0114116496 | 0.0115739628 | 0.0117372513 | 0.0119015010 | 0.0120666981 | 0.0122328286 |
| 17 | 0.0108538064 | 0.0110168721 | 0.0111809627 | 0.0113460623 | 0.0115121553 | 0.0116792258 | 0.0118472580 | 0.0120162362 |
| 18 | 0.0106504964 | 0.0108162036 | 0.0109829524 | 0.0111507251 | 0.0113195038 | 0.0114892709 | 0.0116600087 | 0.0118316994 |
| 19 | 0.0104746389 | 0.0106428832 | 0.0108121808 | 0.0109825119 | 0.0111538566 | 0.0113261953 | 0.0114995084 | 0.0116737763 |
| 20 | 0.0103218839 | 0.0104925601 | 0.0106642963 | 0.0108370706 | 0.0110108613 | 0.0111856468 | 0.0113614055 | 0.0115381160 |
| 21 | 0.0101887095 | 0.0103617120 | 0.0105357766 | 0.0107108793 | 0.0108869965 | 0.0110641046 | 0.0112421803 | 0.0114212003 |
| 22 | 0.0100722345 | 0.0102474580 | 0.0104237412 | 0.0106010582 | 0.0107793836 | 0.0109586918 | 0.0111389577 | 0.0113201565 |
| 23 | 0.0099700800 | 0.0101474200 | 0.0103258132 | 0.0105052320 | 0.0106856488 | 0.0108670366 | 0.0110493685 | 0.0112326181 |
| 24 | 0.0098802657 | 0.0100596188 | 0.0102400151 | 0.0104214248 | 0.0106038188 | 0.0107871683 | 0.0109714450 | 0.0111566208 |
| 25 | 0.0098011308 | 0.0099823955 | 0.0101646896 | 0.0103479819 | 0.0105322414 | 0.0107174379 | 0.0109035414 | 0.0110905227 |
| 26 | 0.0097312730 | 0.0099143498 | 0.0100984392 | 0.0102835082 | 0.0104695246 | 0.0106564565 | 0.0108442729 | 0.0110329433 |
| 27 | 0.0096695016 | 0.0098542931 | 0.0100400777 | 0.0102268209 | 0.0104144889 | 0.0106030487 | 0.0107924680 | 0.0109827154 |
| 28 | 0.0096147995 | 0.0098012113 | 0.0099885940 | 0.0101769118 | 0.0103661298 | 0.0105562138 | 0.0107471306 | 0.0109388478 |
| 29 | 0.0095662943 | 0.0097542345 | 0.0099431214 | 0.0101329179 | 0.0103235881 | 0.0105150969 | 0.0107074102 | 0.0109004947 |
| 30 | 0.0095232340 | 0.0097126139 | 0.0099029143 | 0.0100940973 | 0.0102861260 | 0.0104789643 | 0.0106725776 | 0.0108669321 |
13.00% to 14.75%
| Term (years) | 13.00% | 13.25% | 13.50% | 13.75% | 14.00% | 14.25% | 14.50% | 14.75% |
|---|---|---|---|---|---|---|---|---|
| 1 | 0.0893172757 | 0.0894346092 | 0.0895520274 | 0.0896695302 | 0.0897871176 | 0.0899047895 | 0.0900225460 | 0.0901403869 |
| 2 | 0.0475418226 | 0.0476593339 | 0.0477770145 | 0.0478948643 | 0.0480128833 | 0.0481310712 | 0.0482494280 | 0.0483679537 |
| 3 | 0.0336939520 | 0.0338144937 | 0.0339352873 | 0.0340563328 | 0.0341776298 | 0.0342991781 | 0.0344209774 | 0.0345430277 |
| 4 | 0.0268274959 | 0.0269517434 | 0.0270763230 | 0.0272012341 | 0.0273264765 | 0.0274520496 | 0.0275779529 | 0.0277041859 |
| 5 | 0.0227530730 | 0.0228812552 | 0.0230098460 | 0.0231388449 | 0.0232682508 | 0.0233980632 | 0.0235282811 | 0.0236589037 |
| 6 | 0.0200741052 | 0.0202062930 | 0.0203389622 | 0.0204721114 | 0.0206057395 | 0.0207398450 | 0.0208744268 | 0.0210094833 |
| 7 | 0.0181919633 | 0.0183281534 | 0.0184648928 | 0.0186021795 | 0.0187400116 | 0.0188783872 | 0.0190173042 | 0.0191567608 |
| 8 | 0.0168072551 | 0.0169474014 | 0.0170881597 | 0.0172295271 | 0.0173715010 | 0.0175140786 | 0.0176572571 | 0.0178010336 |
| 9 | 0.0157535877 | 0.0158976165 | 0.0160423141 | 0.0161876769 | 0.0163337012 | 0.0164803831 | 0.0166277190 | 0.0167757050 |
| 10 | 0.0149310740 | 0.0150788916 | 0.0152274289 | 0.0153766812 | 0.0155266435 | 0.0156773111 | 0.0158286789 | 0.0159807422 |
| 11 | 0.0142761079 | 0.0144276058 | 0.0145798680 | 0.0147328885 | 0.0148866612 | 0.0150411798 | 0.0151964383 | 0.0153524303 |
| 12 | 0.0137462524 | 0.0139013108 | 0.0140571716 | 0.0142138275 | 0.0143712708 | 0.0145294942 | 0.0146884899 | 0.0148482502 |
| 13 | 0.0133121026 | 0.0134705927 | 0.0136299170 | 0.0137900665 | 0.0139510322 | 0.0141128049 | 0.0142753757 | 0.0144387353 |
| 14 | 0.0129526358 | 0.0131144223 | 0.0132770683 | 0.0134405631 | 0.0136048961 | 0.0137700568 | 0.0139360343 | 0.0141028180 |
| 15 | 0.0126524217 | 0.0128173643 | 0.0129831854 | 0.0131498727 | 0.0133174139 | 0.0134857966 | 0.0136550086 | 0.0138250376 |
| 16 | 0.0123998784 | 0.0125678337 | 0.0127366804 | 0.0129064044 | 0.0130769918 | 0.0132484286 | 0.0134207008 | 0.0135937946 |
| 17 | 0.0121861444 | 0.0123569668 | 0.0125286878 | 0.0127012915 | 0.0128747623 | 0.0130490845 | 0.0132242426 | 0.0134002210 |
| 18 | 0.0120043253 | 0.0121778689 | 0.0123523127 | 0.0125276391 | 0.0127038309 | 0.0128808707 | 0.0130587414 | 0.0132374260 |
| 19 | 0.0118489795 | 0.0120250987 | 0.0122021146 | 0.0123800080 | 0.0125587600 | 0.0127383517 | 0.0129187646 | 0.0130999801 |
| 20 | 0.0117157571 | 0.0118943077 | 0.0120737468 | 0.0122540538 | 0.0124352081 | 0.0126171894 | 0.0127999777 | 0.0129835530 |
| 21 | 0.0116011418 | 0.0117819820 | 0.0119636984 | 0.0121462688 | 0.0123296711 | 0.0125138838 | 0.0126988854 | 0.0128846549 |
| 22 | 0.0115022636 | 0.0116852547 | 0.0118691058 | 0.0120537933 | 0.0122392939 | 0.0124255848 | 0.0126126432 | 0.0128004472 |
| 23 | 0.0114167592 | 0.0116017662 | 0.0117876137 | 0.0119742768 | 0.0121617311 | 0.0123499526 | 0.0125389176 | 0.0127286030 |
| 24 | 0.0113426682 | 0.0115295604 | 0.0117172706 | 0.0119057730 | 0.0120950420 | 0.0122850526 | 0.0124757804 | 0.0126672014 |
| 25 | 0.0112783530 | 0.0114670043 | 0.0116564488 | 0.0118466597 | 0.0120376104 | 0.0122292753 | 0.0124216292 | 0.0126146474 |
| 26 | 0.0112224377 | 0.0114127271 | 0.0116037829 | 0.0117955773 | 0.0119880832 | 0.0121812743 | 0.0123751247 | 0.0125696094 |
| 27 | 0.0111737600 | 0.0113655718 | 0.0115581215 | 0.0117513808 | 0.0119453218 | 0.0121399178 | 0.0123351426 | 0.0125309709 |
| 28 | 0.0111313337 | 0.0113245577 | 0.0115184899 | 0.0117131014 | 0.0119083641 | 0.0121042508 | 0.0123007352 | 0.0124977918 |
| 29 | 0.0110943183 | 0.0112888499 | 0.0114840590 | 0.0116799165 | 0.0118763941 | 0.0120734643 | 0.0122711009 | 0.0124692782 |
| 30 | 0.0110619952 | 0.0112577352 | 0.0114541218 | 0.0116511253 | 0.0118487175 | 0.0120468709 | 0.0122455593 | 0.0124447572 |
15.00% to 16.75%
| Term (years) | 15.00% | 15.25% | 15.50% | 15.75% | 16.00% | 16.25% | 16.50% | 16.75% |
|---|---|---|---|---|---|---|---|---|
| 1 | 0.0902583123 | 0.0903763222 | 0.0904944164 | 0.0906125950 | 0.0907308579 | 0.0908492051 | 0.0909676365 | 0.0910861522 |
| 2 | 0.0484866480 | 0.0486055110 | 0.0487245425 | 0.0488437423 | 0.0489631105 | 0.0490826469 | 0.0492023513 | 0.0493222238 |
| 3 | 0.0346653285 | 0.0347878797 | 0.0349106810 | 0.0350337322 | 0.0351570330 | 0.0352805832 | 0.0354043826 | 0.0355284307 |
| 4 | 0.0278307483 | 0.0279576394 | 0.0280848589 | 0.0282124062 | 0.0283402808 | 0.0284684822 | 0.0285970100 | 0.0287258635 |
| 5 | 0.0237899301 | 0.0239213595 | 0.0240531911 | 0.0241854239 | 0.0243180571 | 0.0244510898 | 0.0245845211 | 0.0247183501 |
| 6 | 0.0211450133 | 0.0212810155 | 0.0214174883 | 0.0215544305 | 0.0216918406 | 0.0218297172 | 0.0219680587 | 0.0221068639 |
| 7 | 0.0192967547 | 0.0194372841 | 0.0195783469 | 0.0197199408 | 0.0198620639 | 0.0200047141 | 0.0201478890 | 0.0202915867 |
| 8 | 0.0179454053 | 0.0180903693 | 0.0182359228 | 0.0183820626 | 0.0185287860 | 0.0186760898 | 0.0188239712 | 0.0189724269 |
| 9 | 0.0169243373 | 0.0170736119 | 0.0172235250 | 0.0173740726 | 0.0175252508 | 0.0176770554 | 0.0178294825 | 0.0179825281 |
| 10 | 0.0161334957 | 0.0162869346 | 0.0164410537 | 0.0165958479 | 0.0167513121 | 0.0169074412 | 0.0170642299 | 0.0172216730 |
| 11 | 0.0155091496 | 0.0156665898 | 0.0158247447 | 0.0159836077 | 0.0161431726 | 0.0163034328 | 0.0164643820 | 0.0166260135 |
| 12 | 0.0150087677 | 0.0151700344 | 0.0153320427 | 0.0154947848 | 0.0156582530 | 0.0158224393 | 0.0159873361 | 0.0161529354 |
| 13 | 0.0146028746 | 0.0147677844 | 0.0149334556 | 0.0150998788 | 0.0152670448 | 0.0154349445 | 0.0156035685 | 0.0157729077 |
| 14 | 0.0142703972 | 0.0144387611 | 0.0146078990 | 0.0147778003 | 0.0149484542 | 0.0151198501 | 0.0152919772 | 0.0154648249 |
| 15 | 0.0139958712 | 0.0141674972 | 0.0143399034 | 0.0145130775 | 0.0146870074 | 0.0148616810 | 0.0150370861 | 0.0152132107 |
| 16 | 0.0137676961 | 0.0139423914 | 0.0141178669 | 0.0142941087 | 0.0144711034 | 0.0146488373 | 0.0148272971 | 0.0150064693 |
| 17 | 0.0135770043 | 0.0137545773 | 0.0139329247 | 0.0141120314 | 0.0142918824 | 0.0144724628 | 0.0146537579 | 0.0148357530 |
| 18 | 0.0134169075 | 0.0135971693 | 0.0137781947 | 0.0139599673 | 0.0141424707 | 0.0143256889 | 0.0145096058 | 0.0146942059 |
| 19 | 0.0132819798 | 0.0134647458 | 0.0136482601 | 0.0138325050 | 0.0140174630 | 0.0142031170 | 0.0143894498 | 0.0145764448 |
| 20 | 0.0131678958 | 0.0133529868 | 0.0135388069 | 0.0137253373 | 0.0139125594 | 0.0141004551 | 0.0142890064 | 0.0144781956 |
| 21 | 0.0130711714 | 0.0132584147 | 0.0134463645 | 0.0136350010 | 0.0138243050 | 0.0140142572 | 0.0142048391 | 0.0143960322 |
| 22 | 0.0129889748 | 0.0131782047 | 0.0133681159 | 0.0135586878 | 0.0137499002 | 0.0139417334 | 0.0141341681 | 0.0143271853 |
| 23 | 0.0129189861 | 0.0131100448 | 0.0133017574 | 0.0134941026 | 0.0136870597 | 0.0138806083 | 0.0140747287 | 0.0142694016 |
| 24 | 0.0128592923 | 0.0130520302 | 0.0132453929 | 0.0134393585 | 0.0136339061 | 0.0138290148 | 0.0140246646 | 0.0142208360 |
| 25 | 0.0128083061 | 0.0130025820 | 0.0131974524 | 0.0133928951 | 0.0135888889 | 0.0137854127 | 0.0139824465 | 0.0141799705 |
| 26 | 0.0127647042 | 0.0129603854 | 0.0131566300 | 0.0133534159 | 0.0135507215 | 0.0137485258 | 0.0139468087 | 0.0141455506 |
| 27 | 0.0127273781 | 0.0129243405 | 0.0131218349 | 0.0133198393 | 0.0135183320 | 0.0137172922 | 0.0139166999 | 0.0141165357 |
| 28 | 0.0126953959 | 0.0128935238 | 0.0130921525 | 0.0132912598 | 0.0134908244 | 0.0136908257 | 0.0138912439 | 0.0140920600 |
| 29 | 0.0126679716 | 0.0128671577 | 0.0130668135 | 0.0132669171 | 0.0134674476 | 0.0136683845 | 0.0138697087 | 0.0140714013 |
| 30 | 0.0126444402 | 0.0128445850 | 0.0130451692 | 0.0132461710 | 0.0134475700 | 0.0136493462 | 0.0138514808 | 0.0140539557 |
17.00% to 18.75%
| Term (years) | 17.00% | 17.25% | 17.50% | 17.75% | 18.00% | 18.25% | 18.50% | 18.75% |
|---|---|---|---|---|---|---|---|---|
| 1 | 0.0912047521 | 0.0913234361 | 0.0914422043 | 0.0915610566 | 0.0916799929 | 0.0917990133 | 0.0919181176 | 0.0920373060 |
| 2 | 0.0494422641 | 0.0495624722 | 0.0496828479 | 0.0498033912 | 0.0499241020 | 0.0500449801 | 0.0501660254 | 0.0502872378 |
| 3 | 0.0356527275 | 0.0357772727 | 0.0359020659 | 0.0360271069 | 0.0361523955 | 0.0362779314 | 0.0364037143 | 0.0365297439 |
| 4 | 0.0288550423 | 0.0289845459 | 0.0291143736 | 0.0292445250 | 0.0293749996 | 0.0295057968 | 0.0296369160 | 0.0297683566 |
| 5 | 0.0248525758 | 0.0249871973 | 0.0251222137 | 0.0252576241 | 0.0253934274 | 0.0255296228 | 0.0256662092 | 0.0258031856 |
| 6 | 0.0222461311 | 0.0223858590 | 0.0225260460 | 0.0226666905 | 0.0228077911 | 0.0229493462 | 0.0230913543 | 0.0232338137 |
| 7 | 0.0204358049 | 0.0205805413 | 0.0207257938 | 0.0208715601 | 0.0210178380 | 0.0211646251 | 0.0213119192 | 0.0214597179 |
| 8 | 0.0191214541 | 0.0192710495 | 0.0194212100 | 0.0195719326 | 0.0197232141 | 0.0198750513 | 0.0200274410 | 0.0201803799 |
| 9 | 0.0181361879 | 0.0182904580 | 0.0184453341 | 0.0186008120 | 0.0187568877 | 0.0189135569 | 0.0190708153 | 0.0192286588 |
| 10 | 0.0173797652 | 0.0175385013 | 0.0176978760 | 0.0178578840 | 0.0180185199 | 0.0181797784 | 0.0183416541 | 0.0185041416 |
| 11 | 0.0167883209 | 0.0169512977 | 0.0171149373 | 0.0172792331 | 0.0174441787 | 0.0176097673 | 0.0177759924 | 0.0179428475 |
| 12 | 0.0163192294 | 0.0164862102 | 0.0166538701 | 0.0168222010 | 0.0169911952 | 0.0171608449 | 0.0173311420 | 0.0175020789 |
| 13 | 0.0159429529 | 0.0161136947 | 0.0162851241 | 0.0164572317 | 0.0166300085 | 0.0168034454 | 0.0169775331 | 0.0171522626 |
| 14 | 0.0156383827 | 0.0158126400 | 0.0159875861 | 0.0161632107 | 0.0163395032 | 0.0165164533 | 0.0166940505 | 0.0168722847 |
| 15 | 0.0153900429 | 0.0155675708 | 0.0157457825 | 0.0159246662 | 0.0161042104 | 0.0162844033 | 0.0164652336 | 0.0166466897 |
| 16 | 0.0151863407 | 0.0153668981 | 0.0155481286 | 0.0157300190 | 0.0159125567 | 0.0160957289 | 0.0162795230 | 0.0164639266 |
| 17 | 0.0150184338 | 0.0152017858 | 0.0153857950 | 0.0155704472 | 0.0157557286 | 0.0159416256 | 0.0161281246 | 0.0163152123 |
| 18 | 0.0148794734 | 0.0150653931 | 0.0152519497 | 0.0154391283 | 0.0156269142 | 0.0158152927 | 0.0160042497 | 0.0161937709 |
| 19 | 0.0147640854 | 0.0149523553 | 0.0151412385 | 0.0153307192 | 0.0155207820 | 0.0157114116 | 0.0159025930 | 0.0160943115 |
| 20 | 0.0146680055 | 0.0148584190 | 0.0150494193 | 0.0152409901 | 0.0154331152 | 0.0156257790 | 0.0158189658 | 0.0160126606 |
| 21 | 0.0145878184 | 0.0147801803 | 0.0149731006 | 0.0151665622 | 0.0153605488 | 0.0155550442 | 0.0157500324 | 0.0159454983 |
| 22 | 0.0145207666 | 0.0147148939 | 0.0149095495 | 0.0151047162 | 0.0153003772 | 0.0154965162 | 0.0156931171 | 0.0158901645 |
| 23 | 0.0144646082 | 0.0146603300 | 0.0148565494 | 0.0150532487 | 0.0152504113 | 0.0154480205 | 0.0156460605 | 0.0158445156 |
| 24 | 0.0144175100 | 0.0146146681 | 0.0148122924 | 0.0150103654 | 0.0152088703 | 0.0154077908 | 0.0156071109 | 0.0158068152 |
| 25 | 0.0143779658 | 0.0145764139 | 0.0147752970 | 0.0149745977 | 0.0151742994 | 0.0153743859 | 0.0155748415 | 0.0157756512 |
| 26 | 0.0143447326 | 0.0145443364 | 0.0147443444 | 0.0149447395 | 0.0151455052 | 0.0153466258 | 0.0155480860 | 0.0157498710 |
| 27 | 0.0143167809 | 0.0145174175 | 0.0147184282 | 0.0149197964 | 0.0151215059 | 0.0153235414 | 0.0155258880 | 0.0157285314 |
| 28 | 0.0142932555 | 0.0144948131 | 0.0146967156 | 0.0148989469 | 0.0151014915 | 0.0153043344 | 0.0155074613 | 0.0157108585 |
| 29 | 0.0142734445 | 0.0144758213 | 0.0146785152 | 0.0148815106 | 0.0150847923 | 0.0152883462 | 0.0154921585 | 0.0156962160 |
| 30 | 0.0142567534 | 0.0144598576 | 0.0146632523 | 0.0148669225 | 0.0150708537 | 0.0152750324 | 0.0154794453 | 0.0156840801 |
19.00% to 20.75%
| Term (years) | 19.00% | 19.25% | 19.50% | 19.75% | 20.00% | 20.25% | 20.50% | 20.75% |
|---|---|---|---|---|---|---|---|---|
| 1 | 0.0921565782 | 0.0922759344 | 0.0923953744 | 0.0925148983 | 0.0926345059 | 0.0927541973 | 0.0928739724 | 0.0929938313 |
| 2 | 0.0504086172 | 0.0505301635 | 0.0506518766 | 0.0507737563 | 0.0508958026 | 0.0510180154 | 0.0511403944 | 0.0512629396 |
| 3 | 0.0366560200 | 0.0367825423 | 0.0369093105 | 0.0370363242 | 0.0371635834 | 0.0372910875 | 0.0374188364 | 0.0375468298 |
| 4 | 0.0299001182 | 0.0300322002 | 0.0301646019 | 0.0302973228 | 0.0304303623 | 0.0305637199 | 0.0306973950 | 0.0308313868 |
| 5 | 0.0259405511 | 0.0260783047 | 0.0262164454 | 0.0263549720 | 0.0264938837 | 0.0266331794 | 0.0267728580 | 0.0269129185 |
| 6 | 0.0233767230 | 0.0235200805 | 0.0236638845 | 0.0238081336 | 0.0239528261 | 0.0240979602 | 0.0242435344 | 0.0243895471 |
| 7 | 0.0216080190 | 0.0217568200 | 0.0219061187 | 0.0220559126 | 0.0222061993 | 0.0223569765 | 0.0225082416 | 0.0226599924 |
| 8 | 0.0203338649 | 0.0204878928 | 0.0206424601 | 0.0207975638 | 0.0209532004 | 0.0211093666 | 0.0212660592 | 0.0214232747 |
| 9 | 0.0193870830 | 0.0195460837 | 0.0197056566 | 0.0198657974 | 0.0200265017 | 0.0201877652 | 0.0203495836 | 0.0205119525 |
| 10 | 0.0186672355 | 0.0188309305 | 0.0189952210 | 0.0191601018 | 0.0193255672 | 0.0194916120 | 0.0196582306 | 0.0198254176 |
| 11 | 0.0181103258 | 0.0182784209 | 0.0184471261 | 0.0186164348 | 0.0187863404 | 0.0189568363 | 0.0191279160 | 0.0192995729 |
| 12 | 0.0176736477 | 0.0178458405 | 0.0180186496 | 0.0181920672 | 0.0183660855 | 0.0185406967 | 0.0187158933 | 0.0188916674 |
| 13 | 0.0173276249 | 0.0175036109 | 0.0176802117 | 0.0178574183 | 0.0180352218 | 0.0182136133 | 0.0183925842 | 0.0185721256 |
| 14 | 0.0170511456 | 0.0172306230 | 0.0174107069 | 0.0175913871 | 0.0177726538 | 0.0179544970 | 0.0181369070 | 0.0183198740 |
| 15 | 0.0168287603 | 0.0170114342 | 0.0171947003 | 0.0173785476 | 0.0175629650 | 0.0177479419 | 0.0179334675 | 0.0181195313 |
| 16 | 0.0166489273 | 0.0168345131 | 0.0170206718 | 0.0172073915 | 0.0173946605 | 0.0175824672 | 0.0177708002 | 0.0179596480 |
| 17 | 0.0165028754 | 0.0166911010 | 0.0168798764 | 0.0170691887 | 0.0172590257 | 0.0174493750 | 0.0176402246 | 0.0178315626 |
| 18 | 0.0163838424 | 0.0165744507 | 0.0167655823 | 0.0169572239 | 0.0171493627 | 0.0173419858 | 0.0175350807 | 0.0177286352 |
| 19 | 0.0162865526 | 0.0164793023 | 0.0166725466 | 0.0168662718 | 0.0170604647 | 0.0172551122 | 0.0174502013 | 0.0176457197 |
| 20 | 0.0162068485 | 0.0164015151 | 0.0165966461 | 0.0167922276 | 0.0169882461 | 0.0171846883 | 0.0173815413 | 0.0175787924 |
| 21 | 0.0161414265 | 0.0163378026 | 0.0165346120 | 0.0167318409 | 0.0169294756 | 0.0171275029 | 0.0173259097 | 0.0175246836 |
| 22 | 0.0160876431 | 0.0162855383 | 0.0164838357 | 0.0166825213 | 0.0168815817 | 0.0170810036 | 0.0172807743 | 0.0174808813 |
| 23 | 0.0160433708 | 0.0162426115 | 0.0164422235 | 0.0166421929 | 0.0168425065 | 0.0170431514 | 0.0172441150 | 0.0174453852 |
| 24 | 0.0160068889 | 0.0162073176 | 0.0164080873 | 0.0166091846 | 0.0168105965 | 0.0170123103 | 0.0172143139 | 0.0174165956 |
| 25 | 0.0159768003 | 0.0161782749 | 0.0163800613 | 0.0165821464 | 0.0167845177 | 0.0169871630 | 0.0171900707 | 0.0173932293 |
| 26 | 0.0159519667 | 0.0161543594 | 0.0163570361 | 0.0165599841 | 0.0167631913 | 0.0169666461 | 0.0171703373 | 0.0173742542 |
| 27 | 0.0159314581 | 0.0161346549 | 0.0163381091 | 0.0165418088 | 0.0167457424 | 0.0169498987 | 0.0171542673 | 0.0173588378 |
| 28 | 0.0159145129 | 0.0161184120 | 0.0163225438 | 0.0165268967 | 0.0167314599 | 0.0169362228 | 0.0171411754 | 0.0173463083 |
| 29 | 0.0159005064 | 0.0161050176 | 0.0163097383 | 0.0165146576 | 0.0167197652 | 0.0169250513 | 0.0171305065 | 0.0173361219 |
| 30 | 0.0158889249 | 0.0160939685 | 0.0162992000 | 0.0165046094 | 0.0167101869 | 0.0169159233 | 0.0171218099 | 0.0173278384 |
Sample computation
Given:
- Home loan amount: 1,000,000.00
- Interest rate: 7.5%
- Term: 20 years
- What will be the monthly amortization?
Step 1 – Find the corresponding amortization factor rate for a 20 year term at 7.5% annual interest rate
The amortization factor rate is 0.0080559319 (see row for 20 years and column for 7.5% of table 7% to 8.75%)
Step 2 – Compute for the monthly amortization
The monthly amortization would be equal to Loan Amount x Amortization Factor Rate
= 1,000,000.00 x 0.0080559319
= 8055.9319
Want a more detailed example?
Click on the following for a more detailed example: How to compute monthly amortization payments
You can also verify the resulting monthly amortization using our home loan calculator.
Can I use this to make an amortization table? Yes you can, but it can be very tedious if you will use excel or google sheets. An easier way is to just use my Amortization Table/Schedule Calculator (With Principal/Interest and more)
~~~
Disclaimer: The data presented above are for reference purposes only. As always, our standard site disclaimer applies.








Thanks, Jay!
I’ve been using the amortization factor table for years.
Wishing you all the best.
– Ace
Great to hear that, thank you Ace. By the way, I just noticed you had an email about inviting me to discuss about foreclosure prevention way back in 2020, which I wasn’t able to read until now. Really sorry about that.:-(
how much is the monthly amortization if i will loan 100000 in 2yrs?what is the computation?example lang po
Ano po interest rate? Kailangan po ang interest rate para macompute
Jay, thank you for these amortization factor tables. I’ve been using it for my real estate deals every single day.
God bless!
Hi Ace, kamusta na? You’re welcome and I’m glad you are using this in your real estate investing. God bless you too!
Hi,
I have one doubt for the factor calculation, could you please advise to me the factor rate will be constant up to maturity up bond. or it will be change monthly basis (like:after repaid payment).
and if factor will be change the difference between previous factor and current factor rate is our this month payment (Previous Factor – Current factor)* Principal amount
Thanks
Good day sir. Example po 235,200 loanable. If 12% interest in 1 year × factor rate 0.0888487887 = 20,897. Which is tama po sa binigay nila sakin computation. Pero ang gusto ko po sana malaman ung monthly if 1 and half year lang po. Di po nila ako mbigyan ng computation.hindi po nila alam paanu icocompute. May factor rate po kayo para sa 1 and half year? I’ll wait for your response po. Thanks po.
how to compute manually the terms of years..??just like 0.0080559319
Hi sir,
Your website is very good and very informative. Without your amortization factor table I wont be able to compute
how did the bank gave me the amount for my monthly payment on my housing loan. I also just found out that my bank gives a higher interest
compared to other banks. I wont mention the name of the bank here but I am quite pissed off w/ them and with the developer as they didnt gave
me other options to choose on w/c bank I want to have for my housing loan be processed.
My question is; pwede ko pa bang ilipat yun housing loan ko po to other banks after 1-2 years. Basically kase nasa kanila yun
title ng house and lot title di ba. So how can I get rid of my present bank to transfer to other bank(w/ lower interest rate) together my loan. 🙂
My friend suggested that I can transfer it to pag-ibig fund but they give a higher/same interest rate.
How bout bank into another bank, is that possible?
Also if you have more time. Please write up that also explains all about the fixed rate and why the intrest goes up depends on number of years.
Thanks and more power.
Hi Sir, your amortization calculator, tables & excel template are very useful but can I still use this for computing the monthly amortization & total loan amount when there are balloon payments made annually for 6 years? Also what if she has a fixed amount only for the monthly payment w/c is lower than the monthly amortization indicated in the excel template? Can I adjust the years of the loan so that the monthly amortization will reflect the amount that she is willing to pay? I tried doing this but am not sure if its correct to adjust the number of years.
You see, my client wants a rent-to-own scheme with 60k/ mo then 400k on the 12 month of every year. How do I compute it? Amount of loan is P5.8M, interest is 6% for 6 years. Thanks in advance.
Hi Maritoni, thanks a lot for the kind words!
Yes, you can also use this for lumpsum payments, but you have to create an amortization schedule, and the principal will adjust accordingly when lumpsum payments are received. How did you arrive at 60K/month?
Hi Jay 🙂 thanks for replying. I’m not really sure how the client arrived at the 60k, I think she felt that it is the only amount she can pay per month. I filled up your excel template & the amortization came out as 96k/mo but since she can only pay 60k/ mo + 400k on the 12th month—do I input those figures on column B (Monthly amortization) instead of the 96k? Was that what you meant when you said the principal will adjust accordingly?
Oh that makes it so much simpler, I was overthinking it. Thanks so much for the clarification 🙂
Yes Maritoni, you can try to enter the 60K in column B, and then you should see the effect on the principal amount, and the enter 400K on the 12th month. After that you need to drag the rows downward to see the rest until the principal becomes zero.
Yes I did that & it worked so well. Thanks again 🙂
Glad to hear that! You’re welcome!
what is the standard formula for monthly amortization for government housing facility?
just wanted to ask. I applied for home loan to Bank A with 6.5% interest fix for 5 years on a 15-year term while from Bank B has 7% interest rate fix for 5 years in 20-year term. Basically looking at these figures, I opted for Bank A. But upon signing the documents, they require bank fees amounting to 88,900 plus annual insurance worth 19k plus. While Bank B only requires 54,431.17 bank charges and 15,438.77 for MRI (insurance) which is optional and is subject to change annually depending on the outstanding balance.
Please advise which has better terms.
Hi sir Jay! how do you compute for the amortization factors in MS excel just like your tables,
Hi Carl, I explained this in the following article, please check it out:
https://www.foreclosurephilippines.com/2009/11/how-to-calculate-for-the-amortization-factor.html
sir saan po malalaman ang mga factore rates halimbawa ng 7 m sa pag ibig for 25 years can you give me a sample computation sir and the site of pag ibig factorate ty
Hi Julie, will get back to you on this. We are also creating an online home loan calculator that uses parameters from Pag-IBIG. We’ll update you all when this is done.
hi po…tanong lng po ako kung pano ang computation ng interest rate ng isang lot sa subdivision na kinuha ko for two years. But sad to say na hinto yung payment ko last January 2012. sopposed to be matatapos sana MAy 9, 2013.
hi sir, can u please post factor rate at 24%, 30% and 32%? thank u very much!
Hi Mhel, okay will add asap…
Hi Mhel, you can now compute for this using the online amortization factor calculator we just created. Please try and let me know what you think. Thanks.
Pingback: Find The Best Financing With Our Home Loan Interest Rates Comparison Chart - ForeclosurePhilippines.com
Pingback: Monthly Amortization Payments: Back to Basics - ForeclosurePhilippines.com
Thanks!
You’re welcome Alex!
Hi, may i ask for the factor rate of 1 and a half year..thank you
hi jes simply divide your total monthly ammort to principal loan ammount to get factor rate. to compute montly ammortization multiply principal ammount into interest rate x term + principal ammount devided by terms.
ex; Pa; 1M * 1.5% = ____ * 18 + 1M / 18 = ____ m.a
for factor rate at 1 and half or 18 mos ; monthly ammort / 1M principal amt = factor rate.
THANKS FOR THE INFO SIR
You’re welcome!
hi
you can give me a factor rate @ 23%
hi you can give factor rate at 23% thanks
hi you have factorate 23%
Hi Joane, you can compute for this using the online amortization factor calculator we just created. Please try and let me know what you think.
Are these amortization factor rates used in the in-house financing of the developers or are these the rates used in the bank financing schemes?
Most probably, unless they use a straight line method or other method.
i just want to know if there’s a law that tells that this is the right formula in any terms of loan..even if u loan from the owner of the house that u’r going to buy?
Hi Jornelle,
I believe there’s no law that imposes the amortization formula to be used for loans. This is just one of the many methods or standards that can be used. I suppose it is up to the lender to decide which method to use. I hope I have answered your question. Thanks for dropping by, cheers!
wow! thank you so much sir this is what i have been looking for yehey!!! 🙂
Thanks!
Excellent update, sir! thanks very much. I have this page bookmarked 🙂 Keep up the good work.
Thanks Jon!
Pingback: How to compute for the monthly amortization payment without a mortgage calculator
How do you compute for the amortization factors. Thanks. Hope to receive reply very soon.
Hi Teresita,
The formula I use is as follows:
Amort factor=I/(1-(1+I)^-M)
where
I = the monthly interest rate which is equal to the annual interest rate divided by 12
M = the loan payment term in months
I use this formula in excel. I believe there is also a built in function in excel that can do this calculation but I haven’t tried that yet.
I got the formula above from my notes when I attended a seminar in real estate investing conducted by Urban Institute of real estate under Engineer Enrico Cruz.
Where is the Urban Institute of Real Estate? How much is the seminar fee?
Hi Cristina, please checkout their website to get more details about Urban Institute of Real Estate using this link: http://www.urban-institute.com/